Case notes: Robots that use motors in the joints present a motion-control challenge. Should the application produce torque using a six-step technique or sinusoidal commutation for brushless dc (BLDC) motors? See diagrams, equations.
A recent consulting project involved selection of electric motors and motor drives for a true articulated joint robot. One major design constraint is that the motors must be physically placed right at the articulated joints, which severely limits the physical size and weight of the motors. A “strong” robot requires maximizing the amount of torque from the embedded small, lightweight electric motors.
As for motor type, a three-phase brushless dc (BLDC) permanent magnet motor with rare earth magnets would appear to be the best choice. Power density is high, and no maintenance should be required unless motor bearings fail. As for the motor drive, since the motors must be operated from a relatively low voltage dc power supply, a traditional three-phase, six transistor MOSFET (metal-oxide semiconductor field-effect transistor) bridge would seem to be the appropriate selection for the power drive circuit. The next design question is to determine the type of motor commutation scheme. Traditional choices would be either six-step (trapezoidal) or sinusoidal (vector control) commutation. The motor is equipped with directly mounted Hall sensors for monitoring rotor position. Hall sensors provide input for a six-step commutation state machine and, since the motor output effectively goes through a substantial speed reduction before engaging the load, the Hall sensor edges, along with a linear interpolation routine, can be used to provide rotor angle feedback with sufficient resolution to drive a sinusoidal algorithm.
Which is the better commutation scheme for this robotic motion-control application? The six-step technique is simple and robust, widely used in many challenging applications. The sinusoidal scheme is more complex, probably requiring a more powerful microcontroller and higher quality, multiphase, motor current sensors.
As stated earlier, this application requires maximizing torque from relatively small motors. Since BLDC motors linear devices, if we commutate the motor properly, output torque will be reasonably proportional to input current. So, the more current we pour in, the more torque we will get out. There are always, of course, limiting factors. Assuming that we can create a drive with as much current generating capability as we want (remember, we are free to mount the drive away from the motor so we do not have a major size constraint), the motor will present a limit on the maximum allowable current in one of two ways.
At some point, as current is increased, we will reach a level where the “permanent” magnets begin to de-magnetize or the motor will reach its maximum operating temperature. If the motor has a good magnetic design and good, strong magnets, then we will reach the maximum continuous current level based on thermal concerns before any concerns about de-magnetizing the rotor.
Given that the motor will operate at its maximum allowable temperature (and maximum allowable internal power dissipation—copper losses), which commutation scheme will deliver more torque? In both cases, the effective thermal resistance from the stator windings to the ambient will be a constant. The greater output torque, at the same level of power loss, would be the correct choice for this application.
The following analysis aims to compare sinusoidal versus six-step commutation for a BLDC motor under certain specific application constraints, producing maximum torque from a given BLDC motor, at relatively low speeds, and with the motor operated at its maximum allowable temperature. The analysis will calculate the expected torque produced as a function of the power dissipated and will show that, if the motor dissipation is held equal in the two cases, then the ratio of the two torque levels produced is constant, and that the sinusoidal method is superior.
Let W = total resistive power dissipation (Watts) for the motor
Let R = line to neutral resistance (Ohms)
Let S = speed in rpm
Let I = six-step current peak (assume flat or dc) in Amps
Let V = peak of sinusoidal BEMF (back electromotive force in volts)
Let P = average power delivered to BEMF (converted to mechanical power) in Watts
Figure 1 depicts an electrical model for motor calculations. Motor inductance is not included because low-speed operation is assumed.
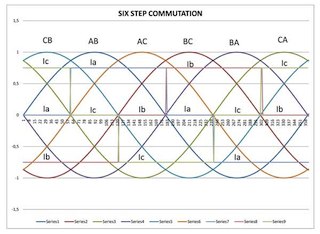
The six-step case in Figure 2 shows motor BEMF and six-step phase currents.
With only two phases conducting at any time, power dissipation in each phase =
Equation 1
Because the situation is symmetrical over the other five steps in a full cycle, there’s only a need to analyze over one 60 degree interval. Apply a flat (dc) current, which flows into phase A and out of phase B. This interval is easily identified in Figure 2 as that in which Ia is positive, Ib is equal in magnitude and negative, and Ic is zero. Apply the current in phase with the phase AB line-to-line BEMF in an interval from 60 degrees (30 degrees before the peak) to 120 degrees (30 degrees after the peak). If we now consider the line-to-neutral BEMF (as Figure 2 shows), the instantaneous power being delivered to the two BEMF is:
Vab is the line-to-line BEMF, which for a three-phase, 120 degree shifted waveform is equal in amplitude to the line-to-neutral amplitude times sqrt (3) so that:
Since the current is constant and the BEMF is a sinusoid, the waveform of instantaneous power will look like a sine wave with an amplitude of: . We know from calculus that the average value of a sine wave over the interval from 60 degrees to 120 degrees is equal to:
This gives us Equation 2:
Substituting EQ1 into EQ2 yields Equation 3:
Let T = average torque in IN-OZ
Let K = 1352.18 = a conversion factor such that:
Thus, the expression for torque in the six-step case is
Equation 4:
SINUSOIDAL CASE:
Let A = RMS value of BEMF (volts line to neutral) = V /
Let B = RMS value of phase current (Amps)
With three phases conducting, power in each phase = W/3
Equation 5:
Assume a unity power factor is maintained, then phase power is BEMF(RMS) times phase current(RMS)
Equation 6:
Substituting for A yields the expression for torque in the sinusoidal case:
Equation 7:
Repeating here, for convenience, the expression for torque in the six-step case is:
Equation 4:
To find the ratio of T (sinusoidal) to T (six-step) multiply T (sinusoidal) by 1/T (six-step)
Conclusion: if we operate a three-phase BLDC motor with a sinusoidal BEMF in a range of speed where only resistive losses are significant (low speed) and load the motor to a fixed level of power dissipation (perhaps that which will produce the maximum allowable temperature rise), then the torque produced by the motor will be 4.72% greater than we would expect operating the motor with traditional six-step commutation under the same power dissipation restraints. This ratio would apply to the average torque in both cases, although the torque in the sinusoidal case would be, theoretically, flat.
– Dennis Nolan is applications engineer, STMicroelectronics, www.st.com. Edited by Mark T. Hoske, CFE Media, Control Engineering, www.controleng.com.
Also see, for related articles, products, and trends…
Machine Control Channel
Motors and Drives Channel